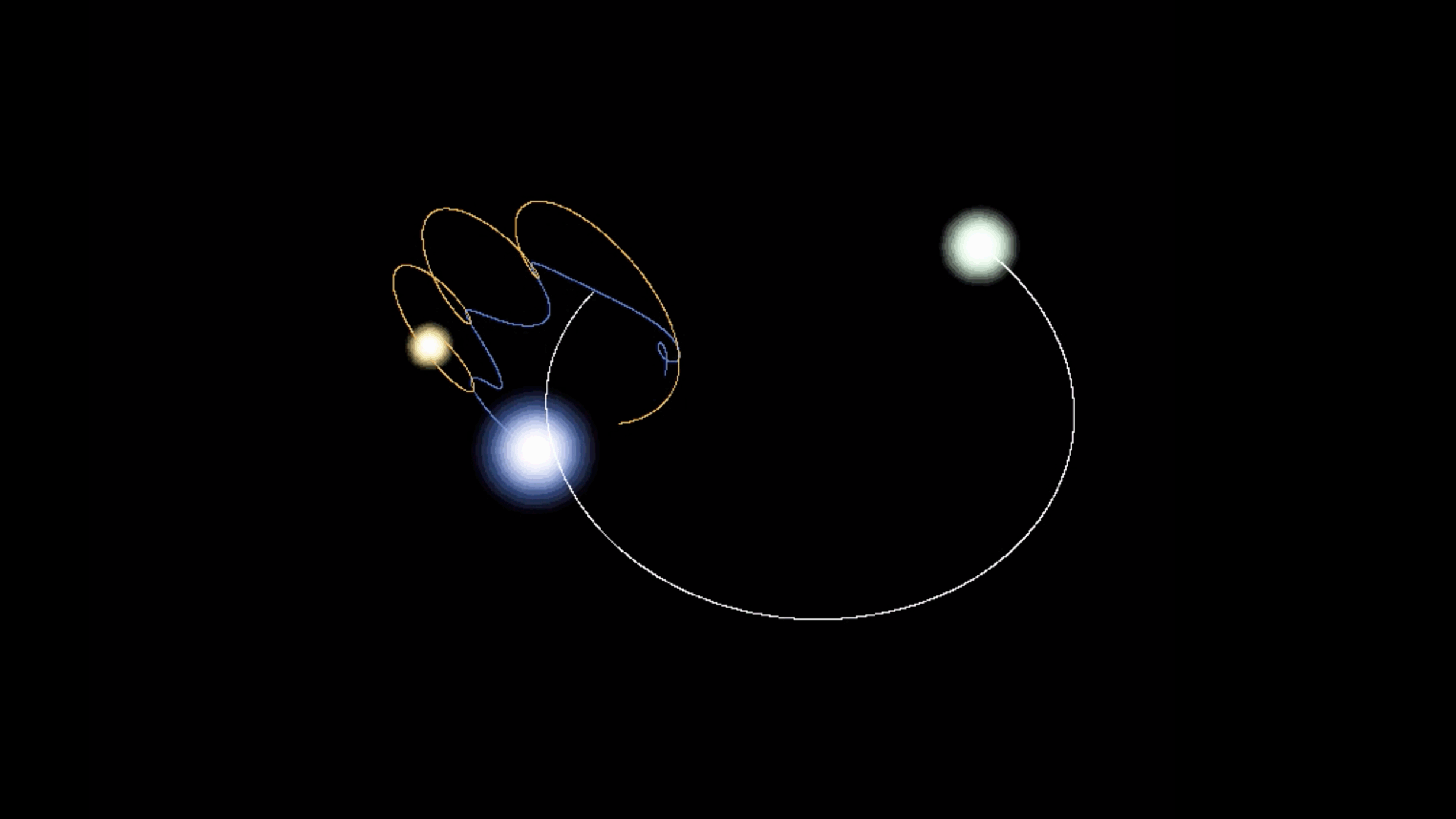
If three objects of comparable size and distance from a center point in space orbit each other, the system is thrown into chaos—one body is always expelled while the other two form a binary system.Courtesy of Aaron Geller, Northwestern University and the Adler Planetarium
Isaac Newton’s ideas laid the foundation for modern physics, but they also uncovered a problem that has stumped scientists for centuries. The three-body problem—the question of how three objects orbit one another—was once considered intractable.
“The three-body problem has a very rich and interesting history,” said Museum Research Associate and former postdoc Nathan Leigh. “At one point, the King of Sweden even offered a prize to any physicist that could solve the three-body problem. The prize was eventually given to Henri Poincaré, who showed that it would be impossible to ever find such a solution.”
When two bodies, or three bodies of different sizes and distances—for example, the Sun, Earth, and Moon—orbit a center point, scientists can easily calculate their movements using Newton's laws of motion. However, if all three objects are of a comparable size and distance from the center point, the whole system is thrown into chaos, making it impossible to track the bodies’ movements using regular math.
The term “chaotic” implies that the system loses all memory of its initial configuration or state, such that the final outcome ends up very sensitive to small perturbations in the initial conditions (i.e., particle positions and/or velocities). This is also called the butterfly effect and can be the difference between wildly different outcomes that come from almost exactly the same initial state for the system.
Leigh, now a professor at Chile’s La Universidad de Concepción, and collaborator Nicholas Stone at the Hebrew University of Jerusalem, have developed a statistical solution for the three-body problem that embraces this chaotic nature. Their findings were recently published in the journal Nature.
Chaotic three-body systems always disintegrate, eventually expelling one member of the trio while the other two remaining bodies form a stable binary system. This relationship was the focus of the new solution, which allows scientists to precisely calculate probabilities for each possible outcome with a “pen-and-paper” method.
“No longer do you have to use a computer to perform millions or billions of three-body problems, each perturbed slightly from some otherwise identical initial state, to figure out how likely each outcome is,” Leigh said.
While the researchers stress that their findings do not represent an exact solution to the three-body problem, they say that statistical solutions are still extremely helpful in that they allow physicists to visualize complicated processes. Triple systems are very common in space, and recent work has shown they are the primary way that black hole mergers—an increasingly popular area of research—are formed.
